6 Fundamental Trig Identities – Trigonometry is a branch of mathematics that deals with the relationships between angles and sides in triangles. It’s a vital component of mathematics education and plays a significant role in fields such as physics, engineering, and computer science.
6 Fundamental Trig Identities
As students embark on their math tuition journeys, understanding trigonometric identities is essential. In this article, we’ll explore 6 fundamental trig identities that serve as the building blocks for solving trigonometric problems.
- Pythagorean Identities
- Reciprocal Identities
- Quotient Identities
- Co-Function Identities
- Even-Odd Identities
- Double-Angle Identities
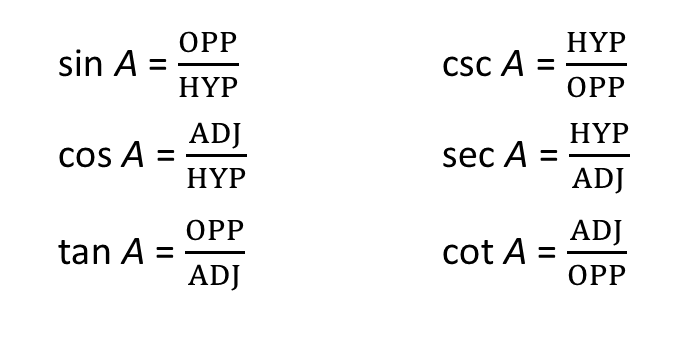
- Pythagorean Identities
One of the most famous and crucial trigonometric identities is the Pythagorean identity. This identity is derived from the Pythagorean theorem, which relates the sides of a right triangle. The Pythagorean identity states that:
sin2(x)+cos2(x)=1sin2(x)+cos2(x)=1
This identity allows us to express any trigonometric function in terms of sine and cosine. It is fundamental in simplifying complex trigonometric expressions and is often used in calculus and physics problems.
- Reciprocal Identities
Reciprocal identities involve the reciprocals of the three primary trigonometric functions: sine, cosine, and tangent. These identities are essential in simplifying expressions and solving trigonometric equations. The reciprocal identities are as follows:
csc(x)=1sin(x)csc(x)=sin(x)1
sec(x)=1cos(x)sec(x)=cos(x)1
cot(x)=1tan(x)cot(x)=tan(x)1
Understanding these identities is crucial for working with trigonometric functions in various contexts.
- Quotient Identities
Quotient identities provide an elegant way to express the three primary trigonometric functions in terms of each other. These identities are particularly useful when you need to simplify expressions or solve trigonometric equations. The quotient identities are as follows:
tan(x)=sin(x)cos(x)tan(x)=cos(x)sin(x)
cot(x)=cos(x)sin(x)cot(x)=sin(x)cos(x)
sec(x)=1cos(x)sec(x)=cos(x)1
csc(x)=1sin(x)csc(x)=sin(x)1
Mastery of these identities allows students to manipulate trigonometric functions effectively.
4 Co-Function Identities
Co-function identities relate complementary angles, which are angles whose sum is 90 degrees (or π/2 radians). These identities are often used in trigonometric applications and calculus. The co-function identities are:
sin(π2−x)=cos(x)sin(2π−x)=cos(x)
cos(π2−x)=sin(x)cos(2π−x)=sin(x)
tan(π2−x)=1tan(x)tan(2π−x)=tan(x)1
Co-function identities are useful when dealing with problems involving angles that are complements of one another.
- Even-Odd Identities
Even-odd identities classify trigonometric functions based on their symmetry. The even functions are symmetric about the y-axis, while the odd functions are antisymmetric about the origin. The even-odd identities for the primary trigonometric functions are:
Even functions:
cos(−x)=cos(x)cos(−x)=cos(x)
sec(−x)=sec(x)sec(−x)=sec(x)
Odd functions:
sin(−x)=−sin(x)sin(−x)=−sin(x)
tan(−x)=−tan(x)tan(−x)=−tan(x)
csc(−x)=−csc(x)csc(−x)=−csc(x)
cot(−x)=−cot(x)cot(−x)=−cot(x)
Understanding these properties helps simplify trigonometric expressions and equations.
- Double-Angle Identities
Double-angle identities are essential when working with angles that are twice the size of a given angle. These identities are particularly useful in calculus, trigonometric integration, and solving trigonometric equations. The primary double-angle identities are:
sin(2x)=2sin(x)cos(x)sin(2x)=2sin(x)cos(x)
cos(2x)=cos2(x)−sin2(x)=2cos2(x)−1=1−2sin2(x)cos(2x)=cos2(x)−sin2(x)=2cos2(x)−1=1−2sin2(x)
Mastery of these identities allows for the simplification of complex trigonometric expressions involving double angles.
Conclusion
As students embark on their math tuition journeys, mastering these 6 fundamental trig identities is essential for success in trigonometry and its applications in various fields. Whether simplifying expressions, solving equations, or tackling calculus problems, a solid understanding of these identities forms the foundation for mathematical success. Trigonometry may seem challenging at first, but with practice and a firm grasp of these identities, students can navigate its intricacies with confidence and skill.
Hope you like this:


