Discover the power of the Reciprocal Function in Trigonometry! This fundamental concept unlocks a new understanding of the relationships between angles and the lengths of sides in triangles. Join us on a journey of exploration and mastery.
Reciprocal Function in Trigonometry
In trigonometry, the reciprocal function refers to the inverse trigonometric functions. These are functions that take an angle as input and produce a ratio of the sides of a right triangle as output.
The six primary inverse trigonometric functions are:
- Arcsine (asin or sin^-1)
- Arccosine (acos or cos^-1)
- Arctangent (atan or tan^-1)
- Arccotangent (acot or cot^-1)
- Arcsecant (asec or sec^-1)
- Arccosecant (acosec or cosec^-1)
Each of these functions is the inverse of one of the six primary trigonometric functions. For example, the arccosine function is the inverse of the cosine function. The inverse functions are useful for solving problems involving triangles, and they play an important role in many areas of mathematics, including calculus and engineering.
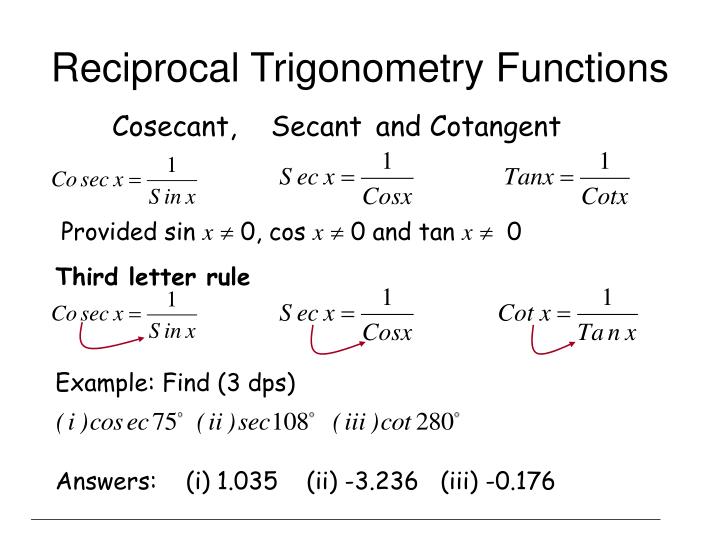
Reciprocal Formula of Trigonometry
The formula for the reciprocal function in trigonometry is given as:
cotθ = 1/tanθ
where cotθ is the reciprocal of tanθ, and θ is the angle in radians.
Examples of Reciprocal Function In Trigonometry
One example of a reciprocal function in trigonometry is the cosecant (csc) and the secant (sec).
Cosecant is defined as 1/sin(x), where sin(x) is the sine function. So, if we want to find the reciprocal of cosecant, we simply switch the denominator and numerator:
csc(x) = 1/sin(x)
• Reciprocal of cosecant = sin(x)/1 = sin(x)
Similarly, secant is defined as 1/cos(x), where cos(x) is the cosine function. To find the reciprocal of secant, we switch the denominator and numerator:
sec(x) = 1/cos(x)
• Reciprocal of secant = cos(x)/1 = cos(x)
So, in this example, cosecant and sine are reciprocal functions, and secant and cosine are reciprocal functions.
In conclusion, there are many examples that you can find in your book, notes, or research paper. In conclusion, the inverse trigonometric functions are referred to as the reciprocal function in trigonometry. Moreover, given the side ratios of a right triangle, these formulas are used to determine the angles in the triangle.

