Trig Reciprocal Identities, trigonometry is a branch of mathematics that studies the relationships between angles and lengths in triangles. The term “reciprocal” refers to the inverse of a function or quantity, meaning that the product of the original quantity and its reciprocal is equal to 1.
Trig Reciprocal Identities
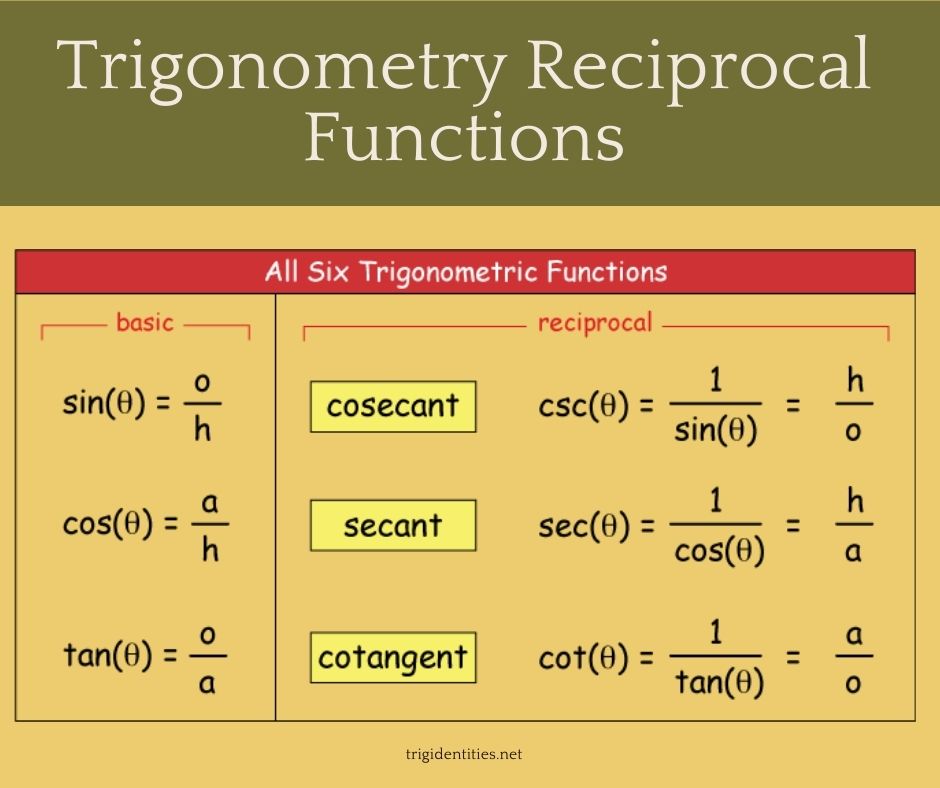
In trigonometry, reciprocal identities important formulas that relate the reciprocals of the six trigonometric functions: sine, cosine, tangent, cosecant, secant, and cotangent.
- One of the most fundamental reciprocal identities is the relationship between sine and cosecant. The sine of an angle is defined as the ratio of the side opposite the angle to the hypotenuse in a right triangle. The cosecant is the reciprocal of the sine, and is defined as the ratio of the hypotenuse to the side opposite the angle. Mathematically, this relationship can expressed as:
csc(θ) = 1/sin(θ) - Another reciprocal identity involves the cosine and secant functions. The cosine of an angle is defined as the ratio of the adjacent side to the hypotenuse in a right triangle, while the secant is the reciprocal of the cosine, equal to the ratio of the hypotenuse to the adjacent side. This relationship can expressed as:
sec(θ) = 1/cos(θ) - The tangent and cotangent functions also reciprocals of each other. The tangent of an angle the ratio of the side opposite the angle to the adjacent side, while the cotangent the reciprocal of the tangent, equal to the ratio of the adjacent side to the side opposite the angle. This relationship can expressed as:
cot(θ) = 1/tan(θ)
These reciprocal identities important in solving problems in trigonometry, as they allow us to find the value of one trigonometric function if we know the value of another. For example, if we know the value of the sine of an angle, we can use the reciprocal identity between sine and cosecant to find the value of the cosecant. Similarly, if we know the value of the tangent, we can use the reciprocal identity between tangent and cotangent to find the value of the cotangent.
Trigonometry Reciprocal Functions
In addition to the reciprocal identities, there also inverse trigonometric functions that allow us to find the angle corresponding to a given value of a trigonometric function. For example, the inverse sine function, denoted by sin^-1, takes a value between -90° and 90° and returns the angle that has a sine equal to that value.
Similarly, the inverse cosine function, denoted by cos^-1, takes a value between -1 and 1 and returns the angle that has a cosine equal to that value. The inverse tangent function, denoted by tan^-1, takes a value and returns the angle that has a tangent equal to that value. These inverse functions important in solving problems in trigonometry, as they allow us to find the angle corresponding to a given value of a trigonometric function.
Reciprocal identities can also used in solving complex problems in trigonometry. For example, if we have an equation involving the tangent of an angle and we need to simplify it, we can use the reciprocal identity between tangent and cotangent to convert the tangent to a cotangent. Check out other related Trigonometry posts:- Trigonometry Ratios, Inverse Trigonometric Function Graph.
Reciprocal of Trigonometric Ratios
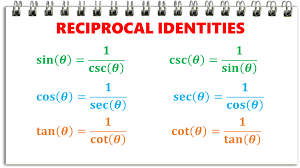
The reciprocal of a trigonometric ratio the multiplicative inverse of the ratio. Here the reciprocals of the primary trigonometric ratios:
-
The Reciprocal of Sine: cscθ = 1 / sinθ
-
Reciprocal of Cosine: secθ = 1 / cosθ
-
Reciprocal of Tangent: cotθ = 1 / tanθ
These reciprocal ratios derived by taking the multiplicative inverse of the corresponding trigonometric ratios. They useful in various trigonometric calculations and identities.
For example, if you have the value of sine (sinθ) for an angle, you can find the reciprocal cosecant (cscθ) by taking the reciprocal of the sine ratio, which = 1 divided by sinθ. Similarly, the reciprocal secant (secθ) obtained by taking 1 divided by the cosine ratio, and the reciprocal cotangent (cotθ) sobtained by taking 1 divided by the tangent ratio.
Reciprocal trigonometric ratios often used in trigonometric identities, solving equations, or simplifying expressions involving trigonometric functions.