Explore the world of Trigonometry and discover how to calculate Limits for Trig Functions! Join us and learn powerful techniques for evaluating limits of trig functions, unlock the ability to solve complex problems with ease, and enhance your mathematical skills today!
Limits For Trig Functions
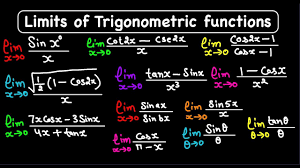
Limits For Trig Functions are functions that describe these relationships in terms of angles. The most common trigonometry functions are sine, cosine, and tangent. In mathematics, limits are an important concept that help us understand the behavior of functions as their inputs approach certain values. The limits of trigonometric functions are essential in calculus and help us understand the behavior of these functions at certain points.
Limits For Trigonometry Functions
The limit of a function is the value that the function approaches as its inputs approach a certain value. In other words, it’s the value that the function gets arbitrarily close to as its inputs get arbitrarily close to a certain value. To calculate the limit of a function, we use the limit formula:
lim(x -> a) f(x) = L
where x approaches a, f(x) is the function, and L is the limit of the function.
The limits of trigonometric functions are important because they help us understand the behavior of these functions at certain points. For example, the limit of the sine function at 0 is 0. This means that as the angle approaches 0, the sine of the angle approaches 0. This is important because it helps us understand the behavior of the sine function as the angle approaches 0.
Limits For Trig Functions – Trig Limits
The limits of the sine, cosine, and tangent functions can be calculated using the limit formula. These limits are important because they help us understand the behavior of these functions at certain points. For example, the limit of the sine function at 0 is 0, the limit of the cosine function at 0 is 1, and the limit of the tangent function at 0 is undefined.
The sine function is defined as the ratio of the side opposite an angle in a right triangle to the hypotenuse. The sine function is periodic, meaning that it repeats every 2π radians. Sine function is also continuous, meaning that it is smooth and has no abrupt changes.
The limit of the sine function at 0 is 0. This means that as the angle approaches 0, the sine of the angle approaches 0. This can be seen by using the limit formula:
lim(x -> 0) sin(x) = 0
The cosine function is defined as the ratio of the adjacent side of an angle in a right triangle to the hypotenuse. The cosine function is also periodic and continuous.
Limit of the cosine function at 0 is 1. This means that as the angle approaches 0, the cosine of the angle approaches 1. This can be seen by using the limit formula:
lim(x -> 0) cos(x) = 1
The tangent function is defined as the ratio of the side opposite an angle to the adjacent side of the angle in a right triangle. The tangent function is not periodic and is not continuous. The tangent function has vertical asymptotes, meaning that it approaches positive or negative infinity at certain angles.
Calculus Trig Limits
The limit of the tangent function at 0 is undefined. This means that as the angle approaches 0, the tangent of the angle does not approach a specific value. This can be seen by using the limit formula:
lim(x -> 0) tan(x) = undefined
In general, the limits of the trigonometric functions help us understand the behavior of these functions at certain points. In calculus, limits for trig functions can be used to determine the behavior of functions as they approach certain values. These limits can help solve problems in real-life applications and are essential in understanding the calculus of trigonometric functions. Practice and understanding of these limits are necessary to excel in calculus.

