Trig Identities Derivatives, inverse functions, and antiderivatives fundamental concepts in calculus and trigonometry. Understanding these topics is essential for solving complex mathematical problems, analyzing functions, and exploring the relationships between trigonometric functions.
Trig Identities Derivatives
Trigonometric identities fundamental equations that establish relationships between the trigonometric functions. When these identities differentiated with respect to a variable, we get the trigonometric derivatives. Understanding trigonometric derivatives is crucial in calculus and various fields of mathematics, physics, and engineering.
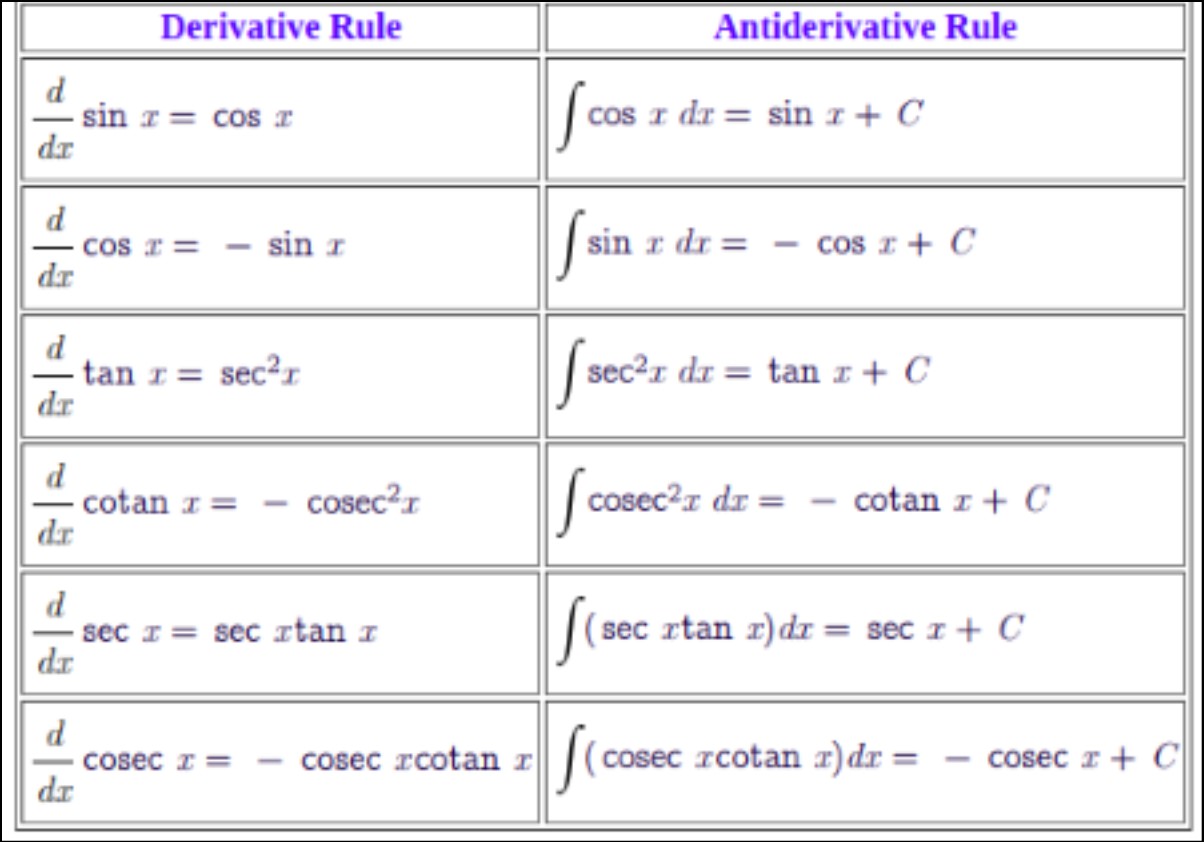
The derivatives of basic trigonometric functions as follows:
- The derivative of sine function (sin x) is cosine function (cos x).
- The derivative of cosine function (cos x) is minus sine function (-sin x).
- The derivative of tangent function (tan x) is secant squared function (sec^2 x).
- The derivative of cotangent function (cot x) is minus cosecant squared function (-csc^2 x).
- The derivative of secant function (sec x) is secant function (sec x) multiplied by tangent function (tan x).
- The derivative of cosecant function (csc x) is minus cosecant function (-csc x) multiplied by cotangent function (cot x).
These derivative rules play a significant role in solving complex calculus problems involving trigonometric functions. By using these identities, we can find rates of change, slopes, and instantaneous velocities of various trigonometric functions. Learn How to Find Trigonometric Ratios with Examples
Antiderivative Trig Identities
Antiderivative, also known as the indefinite integral, is the reverse process of differentiation. It involves finding a function whose derivative matches the given function. Antiderivative trig identities essential in calculus as they allow us to find the original function when the derivative of a trigonometric function is given.
Some common antiderivative trig identities are:
- The antiderivative of cosine function (cos x) is sine function (sin x) + C, where C is the constant of integration.
- The antiderivative of sine function (sin x) is minus cosine function (-cos x) + C.
- The antiderivative of tangent function (tan x) is minus natural logarithm function (-ln|cos x|) + C.
- The antiderivative of cotangent function (cot x) is natural logarithm function (ln|sin x|) + C.
- The antiderivative of secant function (sec x) is natural logarithm function (ln|sec x + tan x|) + C.
- The antiderivative of cosecant function (csc x) is minus natural logarithm function (-ln|csc x + cot x|) + C.
These antiderivative trig identities useful in solving integrals that involve trigonometric functions, and they often require applying additional algebraic techniques and trigonometric substitutions.
Inverse Trig Identities Derivatives
Inverse trigonometric functions provide a way to find angles based on the ratios of sides in a right-angled triangle. When we differentiate inverse trigonometric functions with respect to a variable. We get the derivatives of these functions, which essential in calculus and solving related problems.
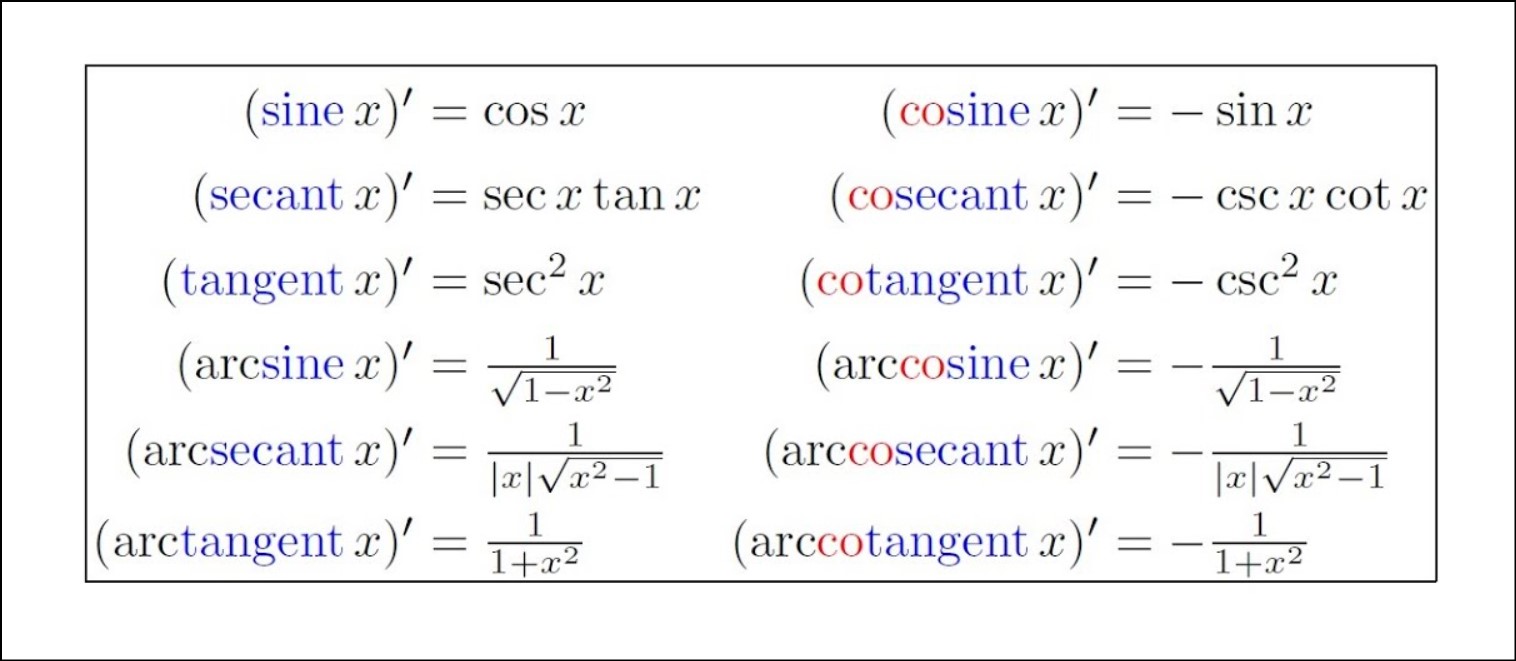
The derivatives of inverse trigonometric functions as follows:
- The derivative of the inverse sine function (arcsin x) is 1 divided by the square root of (1 – x^2).
- The derivative of the inverse cosine function (arccos x) is minus 1 divided by the square root of (1 – x^2).
- The derivative of the inverse tangent function (arctan x) is 1 divided by (1 + x^2).
- The derivative of the inverse cotangent function (arccot x) is minus 1 divided by (1 + x^2).
- The derivative of the inverse secant function (arcsec x) is 1 divided by |x| multiplied by the square root of (x^2 – 1).
- The derivative of the inverse cosecant function (arccsc x) is minus 1 divided by |x| multiplied by the square root of (x^2 – 1).
These derivatives crucial in various calculus applications, including finding slopes of inverse trigonometric functions, rates of change in trigonometric contexts, and solving differential equations involving these functions.